In Part Two George Collin looks at Movement in Perspective.
With the coming of pocket calculators there is a danger that children will not learn how to do anything but the easiest calculations for themselves. In the same way, with developments in computer graphics, there is less incentive for animators to plot perspective. But I would maintain that if you want to be the master of the computer rather than the slave, then you need to understand how to animate in perspective.
There are two basic types of movement to consider:- First where an object moves towards or away from you in a straight line, second where an object rotates.
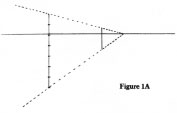 The key to dividing a receding line into equal spaces is the diagonal. Figure 1A shows two uprights, one near and one distant, dotted lines join the bases and tops and indicate the vanishing point on the horizon. The near upright has been divided into seven equal sections.
The key to dividing a receding line into equal spaces is the diagonal. Figure 1A shows two uprights, one near and one distant, dotted lines join the bases and tops and indicate the vanishing point on the horizon. The near upright has been divided into seven equal sections.
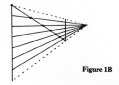 Figure 1B shows those divisions carried to the vanishing point. Now a diagonal is drawn from the top of the near upright to the base of the far one. The points where it crosses the other receding lines are marked.
Figure 1B shows those divisions carried to the vanishing point. Now a diagonal is drawn from the top of the near upright to the base of the far one. The points where it crosses the other receding lines are marked.
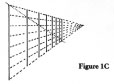 In figure 1C new uprights have been drawn at those crossing points. The receding line is now divided into seven sections evenly spaced in perspective. This method can be used to divide a receding line into any desired number of sections.
In figure 1C new uprights have been drawn at those crossing points. The receding line is now divided into seven sections evenly spaced in perspective. This method can be used to divide a receding line into any desired number of sections.
It may make more sense if you treat the image as the face of a large building with the same number of vertical and horizontal divisions – a diagonal line must line up with the vertical and horizontal intersections. In this sense space and time are interchangeable.
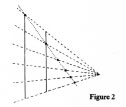 In figure 2 I have assumed that you know where you want the near upright to be and where the vanishing point is and that you want five more positions of the upright receding. Divide the near upright into five and decide how far away the second upright should be. Draw the diagonal from the top of the near upright through the intersection of the second upright and the second receding line. Continue the diagonal until it reaches the bottom receding line. You will see that the positions of the other uprights have been plotted. What these receding lines and uprights represent is up to your imagination. They could be telegraph poles flashing by as you drive along or the key positions of a character walking away. Turn the page on its side and they represent something moving on the ground or the ceiling. For smooth animation you will need to use many more sub-divisions that I have shown. Using the terms from my previous article, figures 1 and 2 illustrate oblique perspective. In aerial perspective the uprights are not parallel. In general terms oblique perspective will suffice so long as the horizon is lower than the top of the near upright. Equal space division in aerial perspective is even more fascinating but is really too long winded for a general article such as this.
In figure 2 I have assumed that you know where you want the near upright to be and where the vanishing point is and that you want five more positions of the upright receding. Divide the near upright into five and decide how far away the second upright should be. Draw the diagonal from the top of the near upright through the intersection of the second upright and the second receding line. Continue the diagonal until it reaches the bottom receding line. You will see that the positions of the other uprights have been plotted. What these receding lines and uprights represent is up to your imagination. They could be telegraph poles flashing by as you drive along or the key positions of a character walking away. Turn the page on its side and they represent something moving on the ground or the ceiling. For smooth animation you will need to use many more sub-divisions that I have shown. Using the terms from my previous article, figures 1 and 2 illustrate oblique perspective. In aerial perspective the uprights are not parallel. In general terms oblique perspective will suffice so long as the horizon is lower than the top of the near upright. Equal space division in aerial perspective is even more fascinating but is really too long winded for a general article such as this.
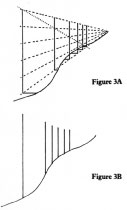 In figures 1 and 2 we were dealing with movement in a straight line. We can adapt the principle to deal with a slight curve. Figure 3A is similar to Figure 1C except that we have superimposed a curved line, perhaps representing a bend in the road. All we have to do is to carry each upright across from the original straight line to the curve. The result is shown in figure 3B. If we were to treat the curve as representing a rise and fall in the road, then the same principle is applied but we move the uprights up or down, not across. Again turn the page on its side to see other applications. For instance, you can use this method to plot the width of a road which curves or rises or does both.
In figures 1 and 2 we were dealing with movement in a straight line. We can adapt the principle to deal with a slight curve. Figure 3A is similar to Figure 1C except that we have superimposed a curved line, perhaps representing a bend in the road. All we have to do is to carry each upright across from the original straight line to the curve. The result is shown in figure 3B. If we were to treat the curve as representing a rise and fall in the road, then the same principle is applied but we move the uprights up or down, not across. Again turn the page on its side to see other applications. For instance, you can use this method to plot the width of a road which curves or rises or does both.
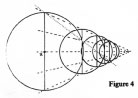 Imagine that you want to show a football coming straight at the camera. The large circle in figure 4 represents the diameter of the ball just before it fills the screen. We now plot a vanishing point in the same way as before, use the method in figures 1 or 2 to plot the diameters of the ball in the frames leading up to it. But in preparing the actual animation drawings, we centre each circle on point X, so that the ball is seen to come straight at us. You will see that we could have placed the vanishing point anywhere on the page which left us enough room to work in. This method can be applied to anything coming straight toward us or away from us.
Imagine that you want to show a football coming straight at the camera. The large circle in figure 4 represents the diameter of the ball just before it fills the screen. We now plot a vanishing point in the same way as before, use the method in figures 1 or 2 to plot the diameters of the ball in the frames leading up to it. But in preparing the actual animation drawings, we centre each circle on point X, so that the ball is seen to come straight at us. You will see that we could have placed the vanishing point anywhere on the page which left us enough room to work in. This method can be applied to anything coming straight toward us or away from us.
Throughout this article I am dealing with movement at a constant speed. If you want to show something moving from rest or slowing down, you will have to adapt these ideas. But at least you will have a basic speed to work from.
page 1 | page 2
